Root Mean Square Velocity Calculator

Gas:

Temperature:

Molar Mass:

Root Mean Square Velocity (m/s): Root Mean Square Velocity (km/s): Root Mean Square Velocity (mph): Root Mean Square Velocity (ft/s): Root Mean Square Velocity (Hz):

1. What is a Root Mean Square Velocity Calculator?

Definition: This calculator computes the root mean square (RMS) velocity (\(v_{rms}\)), the average speed of gas particles in an ideal gas, based on temperature and molar mass.

Purpose: It is used in thermodynamics, statistical mechanics, and chemistry to analyze gas particle motion, aiding in understanding gas behavior, diffusion, and pressure.

2. How Does the Calculator Work?

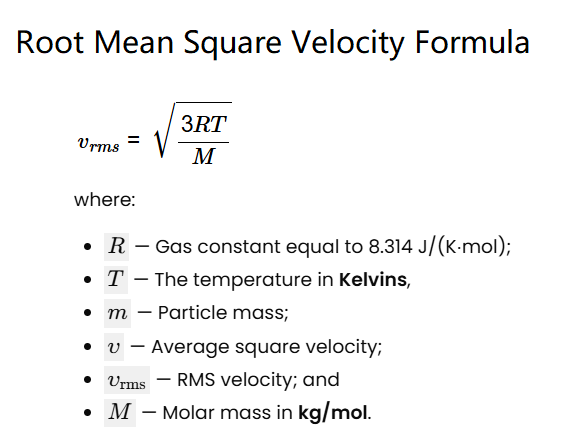
The calculator uses this formula:

\[ v_{rms} = \sqrt{\frac{3RT}{M}} \]

Explanation: Select a gas or enter temperature and molar mass in your chosen units. The calculator converts to base units (K, kg/mol) and outputs RMS velocity in m/s, km/s, mph, ft/s, and Hz.

Unit Conversions:

1 g/mol = 0.001 kg/mol
1 m/s = 0.001 km/s = 2.23694 mph = 3.28084 ft/s
1 m/s = \(\frac{1}{2\pi}\) Hz (for frequency context)

3. Importance of Root Mean Square Velocity

Details: RMS velocity is key to gas kinetics and thermodynamics. Examples include:

Calculating gas pressure via the kinetic theory: \( P = \frac{1}{3} \rho v_{rms}^2 \).
Understanding diffusion rates in gases like oxygen or methane.
Modeling molecular collisions in statistical mechanics.

Applications: Essential for gas laws, atmospheric science, and chemical engineering.

4. Using the Calculator

Tips: Select a gas to auto-fill molar mass or enter custom values with positive values and up to 4 decimal places. Results are in m/s, km/s, mph, ft/s, and Hz. Values < 0.0001 use scientific notation. Avoid zero temperature or molar mass.

Example: For oxygen gas (\(M = 32 \, \text{g/mol} = 0.032 \, \text{kg/mol}\), \(T = 293 \, \text{K}\)):

\(v_{rms} = \sqrt{\frac{3 \times 8.314 \times 293}{0.032}} \approx 483.6 \, \text{m/s}\)
\(v_{rms} \approx 0.4836 \, \text{km/s}\)
\(v_{rms} \approx 1081.8960 \, \text{mph}\)
\(v_{rms} \approx 1587.0489 \, \text{ft/s}\)
\(v_{rms} \approx 76.9755 \, \text{Hz}\)

5. Related Concepts

Average Velocity: \(v_{avg} = \sqrt{\frac{8RT}{\pi M}}\), slightly less than \(v_{rms}\).

Most Probable Velocity: \(v_{mp} = \sqrt{\frac{2RT}{M}}\), the peak of the Maxwell-Boltzmann distribution.

Kinetic Theory of Gases: Relates \(v_{rms}\) to pressure, temperature, and density via \( P = \frac{1}{3} \rho v_{rms}^2 \).

6. Frequently Asked Questions (FAQ)

Q: What’s the difference between \(v_{rms}\), \(v_{avg}\), and \(v_{mp}\)?
A: \(v_{rms}\) is the root mean square (highest), \(v_{avg}\) is the average, and \(v_{mp}\) is the most probable velocity, all from the Maxwell-Boltzmann distribution.

Q: Can temperature or molar mass be negative?
A: No, both must be positive for physical meaning.

Q: Why does the result show zero?
A: If temperature or molar mass is zero, results default to zero.

Q: Why are some results in scientific notation?
A: Values < 0.0001 are displayed as, e.g., \(1.23 \times 10^{-5}\), for clarity.

Q: What does Hz mean here?
A: Hz is included as \( v_{rms} / 2\pi \), representing frequency (non-standard but per request), assuming a cyclical context.

Q: How do I use the gas dropdown?
A: Select a gas to auto-fill molar mass in g/mol, or enter a custom value in kg/mol or g/mol.