HVAC CFM Calculator by Heat Loss

Air Flow Rate (m³/s):

Air Flow Rate (m³/h):

Air Flow Rate (L/s):

What is Air Flow Rate in a Heating System

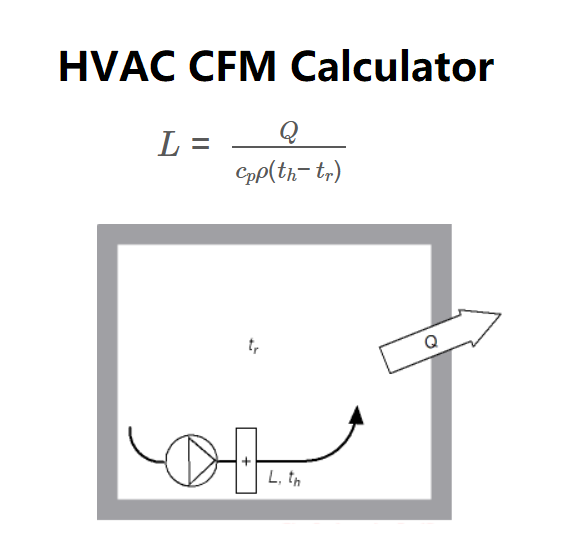
Air flow rate (\( L \)) is the volume of air that must be supplied per second to a heating system to compensate for a given heat loss (\( Q \)). It depends on the temperature difference between the heating air (\( t_h \)) and the room (\( t_r \)), as well as the air's specific heat (\( c_p \)) and density (\( \rho \)). This calculation is essential for designing efficient heating, ventilation, and air conditioning (HVAC) systems.

For example, if a room has a heat loss of 10 kW, a heating air temperature of 40°C, and a room temperature of 20°C, the air flow rate is approximately 0.4149 m³/s.

How to Calculate Air Flow Rate

Formula:

\( L = \frac{Q}{c_p \rho (t_h - t_r)} \)

Where:

\( L \) = Air flow rate (m³/s)
\( Q \) = Heat loss (converted to kW)
\( c_p \) = Specific heat of air = 1.005 kJ/(kg°C)
\( \rho \) = Density of air = 1.2 kg/m³
\( t_h \) = Heating air temperature (converted to °C)
\( t_r \) = Room temperature (converted to °C)

Example Calculation: For a heating system:

Heat Loss (\( Q \)) = 34100 W (converted to 34.1 kW)
Heating Air Temperature (\( t_h \)) = 104°F (converted to 40°C)
Room Temperature (\( t_r \)) = 68°F (converted to 20°C)
Air Flow Rate (\( L \)) = \( \frac{34.1}{1.005 \times 1.2 \times (40 - 20)} \approx 1.4149 \, \text{m}^3/\text{s} \)
In m³/h: \( 1.4149 \times 3600 \approx 5093.64 \, \text{m}^3/\text{h} \)
In L/s: \( 1.4149 \times 1000 \approx 1414.9 \, \text{L}/\text{s} \)

Use the form above to input heat loss, heating air temperature, and room temperature with their respective units to calculate the required air flow rate.

Constants and Conversions Used

The following constants and conversions are used in the calculation:

Parameter	Value
Specific Heat of Air (\( c_p \))	1.005 kJ/(kg°C)
Density of Air (\( \rho \))	1.2 kg/m³
Heat Loss Conversions to kW	W: 0.001, kW: 1.0, hp: 0.7457, Btu/hr: 0.000293071
Temperature Conversions to °C	°F: (°F - 32) × 5/9, K: K - 273.15

Air Flow Rate Units and Conversions

The calculator displays results in the following units:

Air Flow Rate:
- Cubic Meters per Second (m³/s): Base unit
- Cubic Meters per Hour (m³/h): 1 m³/s = 3600 m³/h
- Liters per Second (L/s): 1 m³/s = 1000 L/s

Results are provided in all listed units after calculation.

FAQs

How do you calculate air flow rate for a heating system?

To calculate air flow rate:

Input the heat loss (\( Q \)) in W, kW, hp, or Btu/hr.
Input the heating air temperature (\( t_h \)) and room temperature (\( t_r \)) in °C, °F, or K.
The calculator converts all inputs to kW and °C, then uses the formula \( L = \frac{Q}{c_p \rho (t_h - t_r)} \), with \( c_p = 1.005 \, \text{kJ}/(\text{kg°C}) \) and \( \rho = 1.2 \, \text{kg}/\text{m}^3 \), to compute the air flow rate in m³/s, then converts to m³/h and L/s.

What is the air flow rate for a 50000 Btu/hr heat loss with a heating temperature of 95°F and a room temperature of 65°F?

For \( Q = 50000 \, \text{Btu/hr} \) (converted to 14.65 kW), \( t_h = 95 \, \text{°F} \) (converted to 35°C), \( t_r = 65 \, \text{°F} \) (converted to 18.33°C):

Air Flow Rate (\( L \)) = \( \frac{14.65}{1.005 \times 1.2 \times (35 - 18.33)} \approx 0.607 \, \text{m}^3/\text{s} \)
In m³/h: \( 0.607 \times 3600 \approx 2185.2 \, \text{m}^3/\text{h} \)
In L/s: \( 0.607 \times 1000 \approx 607 \, \text{L}/\text{s} \)