Drift Velocity Calculator

Electric Current:

Number Density of Carriers:

carriers = 10e-6

Cross-Sectional Area:

Charge of Carrier:

Drift Velocity (m/s): Drift Velocity (cm/s): Drift Velocity (μm/s): Drift Velocity (Hz):

1. What is a Drift Velocity Calculator?

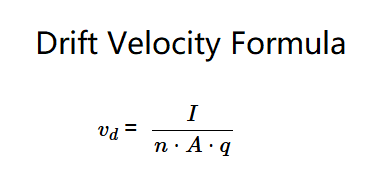
Definition: This calculator computes the drift velocity (\(v_d\)), the average velocity of charge carriers (e.g., electrons) in a material due to an applied electric field.

Purpose: It is used in physics and electrical engineering to analyze the motion of charge carriers in conductors, helping understand electric current and material properties.

2. How Does the Calculator Work?

The calculator uses this formula:

\[ v_d = \frac{I}{n \cdot A \cdot q} \]

Explanation: Input electric current, number density of carriers, cross-sectional area, and charge of the carrier in your chosen units. The calculator converts to base units (A, carriers/m³, m², C) and outputs drift velocity in m/s, cm/s, μm/s, and Hz.

Unit Conversions:

1 carriers/cm³ = \(10^6\) carriers/m³
1 mm² = \(10^{-6}\) m²
1 m/s = 100 cm/s = \(10^6\) μm/s
1 m/s = \(\frac{1}{2\pi}\) Hz (for frequency context)

3. Importance of Drift Velocity

Details: Drift velocity is key to understanding electrical conductivity and current flow. Examples include:

In conductors, it explains why current appears instantaneous despite slow electron drift (e.g., ~23 μm/s in copper).
In semiconductors, it aids in designing transistors and diodes.
In materials science, it helps assess carrier mobility and material efficiency.

Applications: Essential for electronics design, power transmission, and studying material behavior under electric fields.

4. Using the Calculator

Tips: Enter positive values with up to 4 decimal places and select units. Results are in m/s, cm/s, μm/s, and Hz. Values < 0.0001 use scientific notation. Avoid zero density, area, or charge.

Example: For copper with \(I = 1 \, \text{A}\), \(n = 8.5 \times 10^{28} \, \text{carriers/m}^3\), \(A = 3.14 \, \text{mm}^2\), \(q = 1.6 \times 10^{-19} \, \text{C}\):

\(v_d = \frac{1}{(8.5 \times 10^{28}) \times (3.14 \times 10^{-6}) \times (1.6 \times 10^{-19})} \approx 2.34 \times 10^{-5} \, \text{m/s}\)
\(v_d \approx 0.0023 \, \text{cm/s}\)
\(v_d \approx 23.4 \, \text{μm/s}\)
\(v_d \approx 3.722 \times 10^{-6} \, \text{Hz}\)

5. Related Concepts

Current Density: \(J = n \cdot q \cdot v_d\), linking drift velocity to current flow.

Electron Mobility: \(v_d = \mu \cdot E\), where \(\mu\) is mobility and \(E\) is electric field, related to drift velocity.

Thermal Velocity: Random motion of electrons (~1570 km/s in copper), contrasted with slow drift velocity.

6. Frequently Asked Questions (FAQ)

Q: Why is drift velocity so slow compared to current speed?
A: Drift velocity (~μm/s) is slow, but the electric field propagates at nearly light speed, making current appear instantaneous due to many carriers.

Q: Can drift velocity be negative?
A: No, it’s typically positive (magnitude), but direction depends on charge carrier sign (e.g., electrons drift opposite to field).

Q: Why does the result show zero?
A: If density, area, or charge is zero, division by zero occurs, so results default to zero.

Q: Why are some results in scientific notation?
A: Values < 0.0001 are displayed as, e.g., \(1.23 \times 10^{-5}\), for clarity.

Q: What does Hz mean here?
A: Hz is included as \( v_d / 2\pi \), representing frequency (non-standard but per request), assuming a cyclical context.