1. What is a Torsional Constant Calculator?

Definition: This calculator computes the torsional constant ($K$) for various cross-sectional shapes under torsion, using the shape's dimensions.

Purpose: It is used in engineering to analyze how a structural member resists twisting under torque, which is crucial for designing beams, shafts, and other components.

2. How Does the Calculator Work?

The calculator uses the following formulas for $K$, depending on the shape:

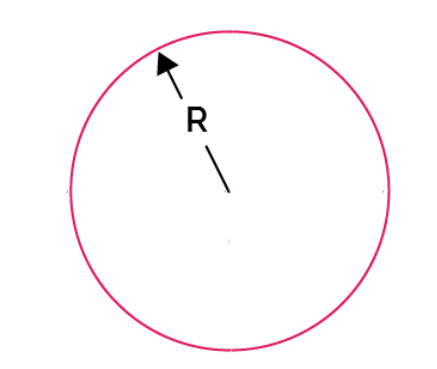
• Circle: $K=\frac{\pi R^4}{2}$
• Solid Ellipse: $K=\frac{\pi a^3{b}^3}{a^2+b^2}$
• Hollow Ellipse: $K=\frac{\pi a^3{b}^3(1-q^4)}{a^2+b^2}$, where $q=\frac{a_n}{a}=\frac{b_n}{b}$
• Thin-Walled Ellipse: $K=\frac{4{\pi }^{2}t(a-\frac{t}{2}{)}^2(b-\frac{t}{2}{)}^2}{U}$, where $U=\pi (a+b-t)t(1+0.258\frac{(a-b{)}^2}{(a+b-t{)}^2})$
• Solid Square: $K=\frac{9a^4}{64}$
• Solid Rectangle: $K=\frac{a^{3}b}{3}-0.214b^4+0.0175\frac{b^8}{a^4}$
• Thin-Walled Rectangle: $K=\frac{2t(a-t{)}^2(b-t{)}^2}{a+b-t-t_1^2-t^2}$
• I-Section: $K=2K_1+K_2+2\alpha D^4$, where $K_1=\frac{a{b}^3}{3}-0.21b^4+0.0175\frac{b^8}{a^4}$, $K_2=\frac{c{d}^3}{3}$, $D=\frac{t_1}{b}(0.15+0.1\frac{r}{b})$, $\alpha =\frac{t_1}{b}(0.15+0.1\frac{r}{b})$, and if $b<d$, then $\frac{t}{t_1}=\frac{b}{d}$, if $d<b$, then $\frac{t}{t_1}=\frac{d}{b}$

Unit Conversions:

• Input Dimensions: m, cm (1 m = 100 cm), mm (1 m = 1000 mm)
• Torsional Constant ($K$): m⁴, cm⁴ (1 m⁴ = 10000 cm⁴), mm⁴ (1 m⁴ = 100000000 mm⁴)

Steps:

• Select a shape to display its formula and input the required dimensions with their units.
• Convert all dimensions to meters for calculation.
• Calculate $K$ based on the shape's formula.
• Convert the result to the selected unit for display, using scientific notation for values less than 0.001, otherwise with 4 decimal places.

3. Importance of Torsional Constant Calculation

Calculating the torsional constant is crucial for:

• Engineering Design: Ensuring structural members can resist twisting under applied torque.
• Material Selection: Choosing shapes with appropriate torsional properties.
• Safety: Preventing torsional failure in mechanical components like shafts and beams.

4. Using the Calculator

Examples:

• Example 1 (Circle): For a circle with $R=10\text{cm}$:
  • Convert: $R=10/100=0.1\text{m}$
  • Torsional Constant: $K=\frac{\pi R^4}{2}=\frac{\pi \times {0.1}^4}{2}=0.0001571{\text{m}}^4$
  • Convert to cm⁴: $K=0.0001571\times 10000=1.571{\text{cm}}^4$
  • Result: $K=1.571{\text{cm}}^4$
• Example 2 (Thin-Walled Rectangle): For a thin-walled rectangle with $a=50\text{mm}$, $b=30\text{mm}$, $t=2\text{mm}$, $t_1=3\text{mm}$:
  • Convert: $a=50/1000=0.05\text{m}$, $b=30/1000=0.03\text{m}$, $t=2/1000=0.002\text{m}$, $t_1=3/1000=0.003\text{m}$
  • Torsional Constant: $K=\frac{2\times 0.002\times (0.05-0.002{)}^2\times (0.03-0.002{)}^2}{0.05+0.03-0.002-{0.003}^2-{0.002}^2}=6.024\times {10}^{-9}{\text{m}}^4$
  • Convert to mm⁴: $K=6.024\times {10}^{-9}\times 100000000=0.6024{\text{mm}}^4$
  • Result: $K=0.6024{\text{mm}}^4$

5. Frequently Asked Questions (FAQ)

Q: What is the torsional constant?
A: The torsional constant ($K$) is a geometric property of a cross-section that quantifies its resistance to torsion.

Q: Why is the torsional constant important?
A: It determines how much a member will twist under torque, which is critical for ensuring structural stability.

Torsional Constant Calculator© - All Rights Reserved 2025