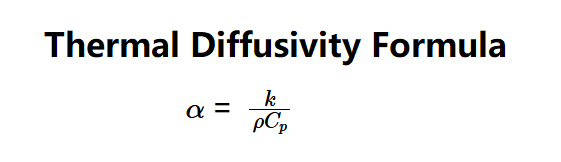Thermal Diffusivity Calculator

Select Material:

Thermal Conductivity (\( k \)):

Density (\( \rho \)):

Specific Heat Capacity (\( C_p \)):

1. What is Thermal Diffusivity Calculator?

Definition: This calculator computes the thermal diffusivity (\( \alpha \)) of a material, which measures how quickly heat diffuses through it, using the formula \( \alpha = \frac{k}{\rho C_p} \).

Purpose: It is used in physics, engineering, and materials science to analyze how fast temperature changes propagate through a material, aiding in the design of thermal systems, insulation, and heat management solutions.

2. How Does the Calculator Work?

The calculator uses the thermal diffusivity formula:

\( \alpha = \frac{k}{\rho C_p} \)

Where:

\( k \): Thermal conductivity (e.g., W/(m·K));
\( \rho \): Density (e.g., kg/m³);
\( C_p \): Specific heat capacity (e.g., J/(kg·K));
\( \alpha \): Thermal diffusivity (e.g., m²/s).

Steps:

Select a material from the list, which automatically fills the thermal conductivity (\( k \)), density (\( \rho \)), and specific heat capacity (\( C_p \)) fields, or choose "Custom" to input your own values.
Edit the values and select their units if needed.
Convert all inputs to base units (W/(m·K) for \( k \), kg/m³ for \( \rho \), J/(kg·K) for \( C_p \)).
Calculate the thermal diffusivity using the formula.
Convert the result to the selected output unit (m²/s, cm²/s, or ft²/s).
Display the result, formatted in scientific notation if the absolute value is less than 0.001, otherwise with 4 decimal places.

3. Importance of Thermal Diffusivity Calculation

Calculating thermal diffusivity is crucial for:

Heat Transfer Analysis: Understanding how quickly heat spreads through a material, which is essential for designing heat sinks, insulators, and thermal barriers.
Material Selection: Choosing materials with appropriate thermal properties for applications like electronics cooling, building insulation, and industrial processes.
Thermal Management: Predicting temperature distribution in transient heat conduction scenarios, such as in engines or manufacturing processes.

4. Using the Calculator

Example 1 (Rubber): Calculate the thermal diffusivity of rubber:

Material: Rubber (Solid);
Thermal Conductivity: \( k = 0.13 \, \text{W/(m·K)} \);
Density: \( \rho = 1,100 \, \text{kg/m³} \);
Specific Heat Capacity: \( C_p = 1,300 \, \text{J/(kg·K)} \);
Thermal Diffusivity: \( \alpha = \frac{0.13}{1,100 \times 1,300} = \frac{0.13}{1,430,000} \approx 9.0909 \times 10^{-8} \, \text{m²/s} \);
Result: \( \alpha = 9.0909 \times 10^{-8} \, \text{m²/s} \).

Example 2 (Custom Values with Different Units): Calculate the thermal diffusivity with custom values:

Thermal Conductivity: \( k = 0.1 \, \text{BTU/(h·ft·°F)} \);
Density: \( \rho = 1 \, \text{g/cm³} \);
Specific Heat Capacity: \( C_p = 1 \, \text{cal/g·K} \);
Convert units: \( k = 0.1 \times 1.730735 = 0.1730735 \, \text{W/(m·K)} \), \( \rho = 1 \times 1000 = 1000 \, \text{kg/m³} \), \( C_p = 1 \times 4186 = 4186 \, \text{J/(kg·K)} \);
Thermal Diffusivity: \( \alpha = \frac{0.1730735}{1000 \times 4186} \approx 4.134 \times 10^{-8} \, \text{m²/s} \);
Result in cm²/s: \( \alpha = 4.134 \times 10^{-8} \times 10000 \approx 4.134 \times 10^{-4} \, \text{cm²/s} \).

5. Frequently Asked Questions (FAQ)

Q: What is thermal diffusivity?
A: Thermal diffusivity (\( \alpha \)) measures how quickly heat diffuses through a material, determined by its thermal conductivity, density, and specific heat capacity, with units of m²/s.

Q: What does volumetric heat capacity mean?
A: Volumetric heat capacity (\( \rho C_p \)) is the heat required to raise the temperature of a unit volume of material by 1 K, with units of J/(m³·K).

Q: Why can I edit the values after selecting a material?
A: Allowing edits after selection provides flexibility, as the predefined values may need adjustment for specific conditions or user requirements.