1. What is a Stress and Strain Calculator?

Definition: This calculator computes the stress (\( \sigma \)), strain (\( \varepsilon \)), and Young’s modulus (\( E \)) of a material based on the applied force, cross-sectional area, and change in length.

Purpose: It is used in engineering and physics to analyze how materials deform under force, which is crucial for designing structures and understanding material properties.

2. How Does the Calculator Work?

The calculator uses the following formulas:

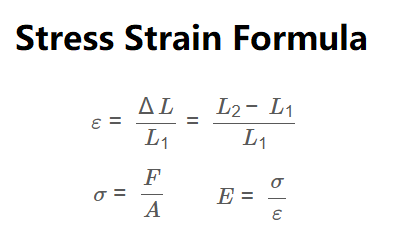
Strain: \[ \varepsilon = \frac{\Delta L}{L_1} = \frac{L_2 - L_1}{L_1} \] Stress: \[ \sigma = \frac{F}{A} \] Young’s Modulus: \[ E = \frac{\sigma}{\varepsilon} \] Where:

• \( \varepsilon \): Strain (dimensionless)
• \( \sigma \): Stress (Pa, kPa, MPa, GPa, psi)
• \( E \): Young’s modulus (Pa, kPa, MPa, GPa, psi)
• \( F \): Force (N, kN, MN, lbf)
• \( A \): Cross-sectional area (mm², cm², m², in², ft²)
• \( L_1 \): Initial length (mm, cm, m, in, ft)
• \( L_2 \): Final length (mm, cm, m, in, ft)

Unit Conversions:

• Force (\( F \)): N, kN (1 kN = 1000 N), MN (1 MN = 1000000 N), lbf (1 lbf = 4.44822 N)
• Area (\( A \)): mm² (1 mm² = 0.000001 m²), cm² (1 cm² = 0.0001 m²), m², in² (1 in² = 0.00064516 m²), ft² (1 ft² = 0.092903 m²)
• Length (\( L_1, L_2 \)): mm (1 mm = 0.001 m), cm (1 cm = 0.01 m), m, in (1 in = 0.0254 m), ft (1 ft = 0.3048 m)
• Stress (\( \sigma \)) and Young’s Modulus (\( E \)): Pa, kPa (1 kPa = 1000 Pa), MPa (1 MPa = 1000000 Pa), GPa (1 GPa = 1000000000 Pa), psi (1 Pa = 0.000145038 psi)

Steps:

• Enter the force, area, initial length, and final length, and select their units.
• Convert all inputs to base units (N for force, m² for area, m for length).
• Calculate strain using \( \varepsilon = \frac{L_2 - L_1}{L_1} \).
• Calculate stress using \( \sigma = \frac{F}{A} \).
• Calculate Young’s modulus using \( E = \frac{\sigma}{\varepsilon} \).
• Convert stress and Young’s modulus to the selected units (Pa, kPa, MPa, GPa, psi).
• Display the results, using scientific notation for values less than 0.001, otherwise with 4 decimal places.

3. Importance of Stress and Strain Calculation

Calculating stress and strain is crucial for:

• Engineering Design: Ensuring materials can withstand applied forces without failing.
• Material Science: Understanding the mechanical properties of materials, such as elasticity and strength.
• Safety: Preventing structural failures in buildings, bridges, and machinery.

4. Using the Calculator

Examples:

• Example 1: For \( F = 5000 \, \text{N} \), \( A = 100 \, \text{mm}^2 \), \( L_1 = 1 \, \text{m} \), \( L_2 = 1.002 \, \text{m} \), stress in MPa, Young’s modulus in GPa:
  • Convert: \( A = 100 \times 0.000001 = 0.0001 \, \text{m}^2 \)
  • Strain: \( \varepsilon = \frac{1.002 - 1}{1} = 0.0020 \)
  • Stress: \( \sigma = \frac{5000}{0.0001} = 50000000 \, \text{Pa} \)
  • Convert to MPa: \( \sigma = 50000000 \div 1000000 = 50.0000 \, \text{MPa} \)
  • Young’s Modulus: \( E = \frac{50000000}{0.002} = 25000000000 \, \text{Pa} \)
  • Convert to GPa: \( E = 25000000000 \div 1000000000 = 25.0000 \, \text{GPa} \)
• Example 2: For \( F = 1000 \, \text{lbf} \), \( A = 2 \, \text{in}^2 \), \( L_1 = 10 \, \text{in} \), \( L_2 = 10.1 \, \text{in} \), stress in psi, Young’s modulus in psi:
  • Convert: \( F = 1000 \times 4.44822 = 4448.22 \, \text{N} \), \( A = 2 \times 0.00064516 = 0.00129032 \, \text{m}^2 \), \( L_1 = 10 \times 0.0254 = 0.254 \, \text{m} \), \( L_2 = 10.1 \times 0.0254 = 0.25654 \, \text{m} \)
  • Strain: \( \varepsilon = \frac{0.25654 - 0.254}{0.254} = 0.0100 \)
  • Stress: \( \sigma = \frac{4448.22}{0.00129032} = 3448275.86 \, \text{Pa} \)
  • Convert to psi: \( \sigma = 3448275.86 \times 0.000145038 = 500.0000 \, \text{psi} \)
  • Young’s Modulus: \( E = \frac{3448275.86}{0.01} = 344827586 \, \text{Pa} \)
  • Convert to psi: \( E = 344827586 \times 0.000145038 = 50000.0000 \, \text{psi} \)

5. Frequently Asked Questions (FAQ)

Q: What is strain?
A: Strain is a dimensionless measure of deformation, defined as the change in length divided by the original length.

Q: What is stress?
A: Stress is the force per unit area applied to a material, measured in units like Pascals (Pa) or psi.

Q: What does Young’s modulus tell us?
A: Young’s modulus measures a material’s stiffness. A higher value indicates a stiffer material that deforms less under stress.

Stress and Strain Calculator© - All Rights Reserved 2025