Schwarzschild Radius Calculator

1. What is Schwarzschild Radius Calculator?

Definition: This calculator computes the Schwarzschild radius ( $r_{s}$ ) of a celestial object, which is the radius of the event horizon of a non-rotating black hole, and the gravitational field ( $g$ ) at that radius, based on the object's mass ( $M$ ).

Purpose: It is used in astrophysics to determine the critical radius at which an object would become a black hole if its mass were compressed within that radius, and to understand the gravitational field strength at the event horizon.

2. How Does the Calculator Work?

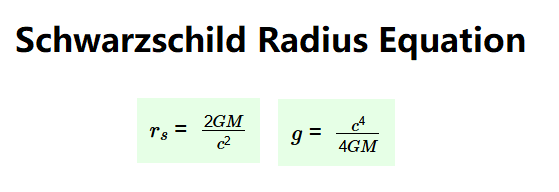
The calculator uses the following equation to compute the Schwarzschild radius:

$r_{s} = \frac{2 G M}{c^{2}}$

The gravitational field at the Schwarzschild radius is calculated using:

$g = \frac{c^{4}}{4 G M}$

Where:

$r_{s}$ : Schwarzschild radius (m)
$g$ : Gravitational field at the Schwarzschild radius (m/s²)
$G$ : Gravitational constant ( $6.67430 \times 10^{- 11} m^{3} {kg}^{- 1} s^{- 2}$ )
$M$ : Mass of the object (kg)
$c$ : Speed of light ( $299, 792, 458 m/s$ )

Unit Conversions:

Mass ( $M$ ):
- 1 kg = 1 kg
- 1 Solar Mass = $1.989 \times 10^{30} kg$
- 1 Earth Mass = $5.972 \times 10^{24} kg$
Schwarzschild Radius ( $r_{s}$ ):
- 1 meter = 1 m
- 1 kilometer = 1000 m
- 1 mile = 1609.344 m
Gravitational Field ( $g$ ):
- 1 m/s² = 1 m/s²
- 1 g = 9.80665 m/s²

Steps:

Enter the mass of the object ( $M$ ) with its respective unit (kg, solar masses, or Earth masses).
Convert the mass to kilograms.
Calculate $r_{s}$ using the Schwarzschild radius equation.
Calculate $g$ at the Schwarzschild radius using the gravitational field equation.
Convert the results to the selected units for display (meters, kilometers, or miles for $r_{s}$ ; m/s² or g for $g$ ).
Display the results with 4 decimal places.

3. Importance of Schwarzschild Radius and Gravitational Field Calculation

Calculating the Schwarzschild radius and gravitational field is crucial for:

Understanding Black Holes: The Schwarzschild radius defines the event horizon of a non-rotating black hole, and the gravitational field at that radius indicates the immense gravitational pull at the boundary.
Astrophysical Research: Helps in studying the properties of black holes, such as their size and the strength of their gravitational effects on surrounding matter.
Space Exploration: Assists in conceptualizing the gravitational effects of massive objects, which is important for planning missions near black holes or other dense objects.

4. Using the Calculator

Example: Calculate the Schwarzschild radius and gravitational field at that radius for the Sun, given its mass $M = 1.989 \times 10^{30} kg$ .

Enter the mass as $1.989 \times 10^{30} kg$ (or select 1 Solar Mass).
The calculator computes:
- $r_{s} = \frac{2 G M}{c^{2}} = \frac{2 \times (6.67430 \times 10^{- 11}) \times (1.989 \times 10^{30})}{(299, 792, 458)^{2}}$
- $r_{s} = \frac{2.657 \times 10^{20}}{8.987 \times 10^{16}} \approx 2953.2501 m$
- In kilometers: $2953.2501 / 1000 \approx 2.9533 km$ , which matches the known Schwarzschild radius of the Sun (approximately 3 km).
- $g = \frac{c^{4}}{4 G M} = \frac{(299, 792, 458)^{4}}{4 \times (6.67430 \times 10^{- 11}) \times (1.989 \times 10^{30})}$
- $g = \frac{8.077 \times 10^{33}}{5.314 \times 10^{20}} \approx 1.520 \times 10^{13} {m/s}^{2}$
- In g: $1.520 \times 10^{13} / 9.80665 \approx 1.550 \times 10^{12} g$ , indicating an extremely strong gravitational field at the event horizon.

5. Frequently Asked Questions (FAQ)

Q: What is the Schwarzschild radius?
A: The Schwarzschild radius ( $r_{s}$ ) is the radius of the event horizon of a non-rotating black hole, the boundary beyond which nothing can escape due to the immense gravitational pull.

Q: What does the gravitational field at the Schwarzschild radius represent?
A: It represents the gravitational acceleration at the event horizon of a black hole, indicating the strength of the gravitational pull at that boundary. For a black hole, this field is extremely large.

Q: Can any object have a Schwarzschild radius?
A: Yes, every object has a theoretical Schwarzschild radius, but only if its mass is compressed within that radius does it become a black hole. For example, Earth's Schwarzschild radius is about 9 mm, but its actual radius is much larger, so it is not a black hole.