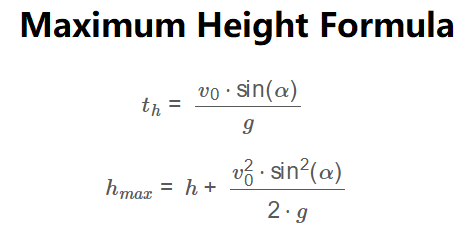Maximum Height Calculator – Projectile Motion calculator with Angle

Velocity (v₀):

Angle of Launch (α):

Initial Height (h):

1. What is a Maximum Height Calculator – Projectile Motion?

Definition: This calculator determines the maximum height reached by a projectile during its flight, given its initial velocity, launch angle, and initial height.

Purpose: It is used in physics to analyze the vertical motion of projectiles, such as a ball thrown at an angle, aiding in understanding projectile motion principles.

2. How Does the Calculator Work?

The calculator uses the following formulas:

Time to Reach Maximum Height: \[ t_h = \frac{v_0 \cdot \sin(\alpha)}{g} \] Maximum Height: \[ h_{max} = h + \frac{v_0^2 \cdot \sin^2(\alpha)}{2 \cdot g} \] Where:

\( h_{max} \): Maximum height (cm, m, in, ft, yd, km, mi)
\( v_0 \): Initial velocity (m/s, km/h, ft/s, mph, kn, ft/min)
\( \alpha \): Angle of launch (degrees, radians)
\( h \): Initial height (cm, m, in, ft, yd, km, mi)
\( g \): Acceleration due to gravity (\( 9.81 \, \text{m/s}^2 \))

Unit Conversions:

Velocity Units (v₀): m/s, km/h, ft/s, mph, kn, ft/min
Angle Units (α): degrees, radians
Height Units (h, h_max): cm, m, in, ft, yd, km, mi

Steps:

Enter the initial velocity (v₀), selecting the unit (m/s, km/h, ft/s, mph, kn, ft/min)
Enter the angle of launch (α), selecting the unit (degrees, radians)
Enter the initial height (h), selecting the unit (cm, m, in, ft, yd, km, mi)
Convert all inputs to SI units (m/s, radians, m)
Calculate the maximum height using the formula
Select the desired unit for the maximum height and view the result

3. Importance of Maximum Height Calculation

Calculating the maximum height of a projectile is crucial for:

Physics Education: Understanding the vertical component of projectile motion.
Sports and Ballistics: Analyzing the trajectory of balls in sports or projectiles in ballistics.
Safety Analysis: Predicting the peak height of objects to ensure safety in scenarios like fireworks or thrown objects.

4. Using the Calculator

Examples:

Example 1: For an initial velocity \( v_0 = 25.36 \, \text{m/s} \), angle \( \alpha = 12^\circ \), initial height \( h = 25 \, \text{m} \):
- Angle in radians = \( 12 \times \frac{\pi}{180} = 0.2094 \, \text{rad} \)
- \( \sin(\alpha) = \sin(0.2094) = 0.2079 \)
- Maximum height contribution = \( \frac{(25.36)^2 \cdot (0.2079)^2}{2 \cdot 9.81} = \frac{643.13 \cdot 0.0432}{19.62} = 1.416 \, \text{m} \)
- Total maximum height = \( 25 + 1.416 = 26.416 \, \text{m} \)
- Displayed as 26.416 m (rounded to 3 decimal places)
Example 2: For an initial velocity \( v_0 = 50 \, \text{mph} \), angle \( \alpha = 45^\circ \), initial height \( h = 10 \, \text{ft} \):
- Velocity in m/s = \( 50 \times 0.44704 = 22.352 \, \text{m/s} \)
- Angle in radians = \( 45 \times \frac{\pi}{180} = 0.7854 \, \text{rad} \)
- Height in m = \( 10 \times 0.3048 = 3.048 \, \text{m} \)
- \( \sin(\alpha) = \sin(0.7854) = 0.7071 \)
- Maximum height contribution = \( \frac{(22.352)^2 \cdot (0.7071)^2}{2 \cdot 9.81} = \frac{499.61 \cdot 0.5}{19.62} = 12.727 \, \text{m} \)
- Total maximum height = \( 3.048 + 12.727 = 15.775 \, \text{m} \)
- In ft = \( 15.775 \times 3.28084 = 51.775 \, \text{ft} \)

5. Frequently Asked Questions (FAQ)

Q: What is the maximum height in projectile motion?
A: The maximum height (\( h_{max} \)) is the highest vertical position a projectile reaches during its trajectory, calculated as \( h_{max} = h + \frac{v_0^2 \cdot \sin^2(\alpha)}{2 \cdot g} \).

Q: Why is the vertical velocity zero at the maximum height?
A: At the maximum height, the projectile stops rising and begins to fall, so its vertical velocity becomes zero (\( v_y = 0 \)).

Q: What happens if the launch angle is 0°?
A: If \( \alpha = 0^\circ \), the projectile moves horizontally, and the maximum height is equal to the initial height (\( h_{max} = h \)).