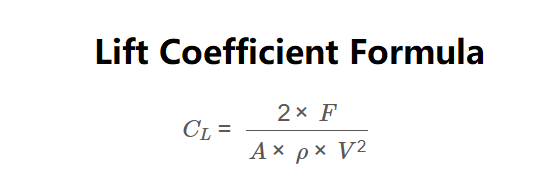Lift Coefficient Calculator

Lift Force (\( F \)):

Surface Area (\( A \)):

Fluid Density (\( \rho \)):

Flow Speed (\( V \)):

1. What is Lift Coefficient Calculator?

Definition: This calculator computes the lift coefficient (\( C_L \)), a dimensionless quantity that quantifies the lift generated by a surface (such as an aircraft wing) relative to the surface area, fluid density, and flow speed.

Purpose: It is used in aerodynamics to evaluate the performance of wings or airfoils, helping aeronautical engineers and RC aircraft enthusiasts estimate lift characteristics for design optimization.

2. How Does the Calculator Work?

The calculator uses the following formula:

Formula: \[ C_L = \frac{2 \times F}{A \times \rho \times V^2} \] Where:

\( C_L \): Lift coefficient (dimensionless)
\( F \): Lift force (N, kN, lbf)
\( A \): Surface area (m², cm², mm², ft², in²)
\( \rho \): Fluid density (kg/m³, g/cm³, kg/cm³, lb/ft³, slug/ft³)
\( V \): Flow speed (m/s, km/h, mph)

Unit Conversions:

Lift Force (\( F \)):
- 1 N = 1 N
- 1 kN = 1000 N
- 1 lbf = 4.44822 N
Surface Area (\( A \)):
- 1 m² = 1 m²
- 1 cm² = 0.0001 m²
- 1 mm² = 0.000001 m²
- 1 ft² = 0.092903 m²
- 1 in² = 0.00064516 m²
Fluid Density (\( \rho \)):
- 1 kg/m³ = 1 kg/m³
- 1 g/cm³ = 1000 kg/m³
- 1 kg/cm³ = 1000000 kg/m³
- 1 lb/ft³ = 16.0185 kg/m³
- 1 slug/ft³ = 515.379 kg/m³
Flow Speed (\( V \)):
- 1 m/s = 1 m/s
- 1 km/h = 0.277778 m/s
- 1 mph = 0.44704 m/s

Steps:

Enter the lift force (\( F \)) with its respective unit (N, kN, lbf).
Enter the surface area (\( A \)) with its respective unit (m², cm², mm², ft², in²).
Enter the fluid density (\( \rho \)) with its respective unit (kg/m³, g/cm³, kg/cm³, lb/ft³, slug/ft³). Default is 1.225 kg/m³ (air at sea level).
Enter the flow speed (\( V \)) with its respective unit (m/s, km/h, mph).
Convert all inputs to SI units (N for force, m² for area, kg/m³ for density, m/s for speed).
Calculate the lift coefficient using \( C_L = \frac{2 \times F}{A \times \rho \times V^2} \).
Display the result, using scientific notation for values less than 0.001, otherwise with 4 decimal places.

3. Importance of Lift Coefficient Calculation

Calculating the lift coefficient is crucial for:

Aircraft Design: Determining the lift capabilities of wings or airfoils, optimizing for efficiency and performance.
Performance Analysis: Estimating how changes in speed, density, or wing area affect lift, aiding in flight planning and safety.
Education and Research: Understanding aerodynamic principles in aerospace engineering and fluid dynamics studies.

4. Using the Calculator

Examples:

Example 1: Calculate the lift coefficient for a wing with a surface area of 1000 cm², moving at a speed of 100 m/s, generating 800 N of lift force, in air with a density of 1.225 kg/m³:
- Enter \( F = 800 \) N.
- Enter \( A = 1000 \) cm².
- Convert to m²: \( A = 1000 \times 0.0001 = 0.1 \, \text{m²} \)
- Enter \( \rho = 1.225 \) kg/m³.
- Enter \( V = 100 \) m/s.
- Calculate denominator: \( A \times \rho \times V^2 = 0.1 \times 1.225 \times 100^2 = 1225 \)
- \( C_L = \frac{2 \times 800}{1225} = \frac{1600}{1225} = 1.3061 \)
- Result: \( C_L = 1.3061 \)
Example 2: Calculate the lift coefficient for a wing with a surface area of 500 in², moving at a speed of 150 mph, generating 2000 lbf of lift force, in air with a density of 0.002378 slug/ft³ (sea level):
- Enter \( F = 2000 \) lbf.
- Convert to N: \( F = 2000 \times 4.44822 = 8896.44 \, \text{N} \)
- Enter \( A = 500 \) in².
- Convert to m²: \( A = 500 \times 0.00064516 = 0.32258 \, \text{m²} \)
- Enter \( \rho = 0.002378 \) slug/ft³.
- Convert to kg/m³: \( \rho = 0.002378 \times 515.379 = 1.2256 \, \text{kg/m³} \)
- Enter \( V = 150 \) mph.
- Convert to m/s: \( V = 150 \times 0.44704 = 67.056 \, \text{m/s} \)
- Calculate denominator: \( A \times \rho \times V^2 = 0.32258 \times 1.2256 \times 67.056^2 = 1778.13 \)
- \( C_L = \frac{2 \times 8896.44}{1778.13} = \frac{17792.88}{1778.13} = 10.0068 \)
- Result: \( C_L = 10.0068 \)

5. Frequently Asked Questions (FAQ)

Q: What factors affect the lift coefficient?
A: The lift coefficient is influenced by the angle of attack, airfoil shape, airspeed, air density, and surface roughness. It also varies with Reynolds number and Mach number, especially at high speeds.

Q: Why is the lift coefficient dimensionless?
A: The lift coefficient normalizes the lift force by the product of area, density, and velocity squared, making it independent of units and allowing comparison across different conditions and designs.

Q: Can the lift coefficient be used for non-aircraft applications?
A: Yes, it can be applied to any lifting surface, such as sails on boats, wind turbine blades, or even sports equipment like golf balls, where lift affects performance.