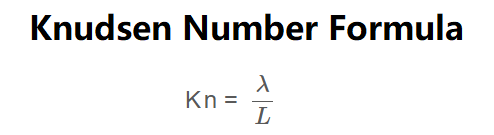Knudsen Number Calculator

Mean Free Path (\( \lambda \)):

Characteristic Linear Dimension (\( L \)):

1. What is Knudsen Number Calculator?

Definition: This calculator computes the Knudsen number (\( \text{Kn} \)), a dimensionless quantity used to determine the flow regime of a gas, based on the mean free path (\( \lambda \)) and the characteristic linear dimension (\( L \)).

Purpose: It is used in fluid dynamics and rarefied gas dynamics to classify flow regimes (continuum, slip, transition, or free molecular flow), which is essential for applications in microfluidics, aerospace, and vacuum technology.

2. How Does the Calculator Work?

The calculator uses the following formula:

Formula: \[ \text{Kn} = \frac{\lambda}{L} \] Where:

\( \text{Kn} \): Knudsen number (dimensionless)
\( \lambda \): Mean free path (m, km, cm, mm, μm, nm, pm, ft, in, mi)
\( L \): Characteristic linear dimension (m, km, cm, mm, μm, ft, in, mi)

Unit Conversions:

Mean Free Path (\( \lambda \)) and Characteristic Linear Dimension (\( L \)):
- 1 m = 1 m
- 1 km = 1000 m
- 1 cm = 0.01 m
- 1 mm = 0.001 m
- 1 μm = 0.000001 m
- 1 nm = 0.000000001 m
- 1 pm = 0.000000000001 m
- 1 ft = 0.3048 m
- 1 in = 0.0254 m
- 1 mi = 1609.34 m
Knudsen Number (\( \text{Kn} \)): Dimensionless, no conversion needed.

Steps:

Enter the mean free path (\( \lambda \)) with its respective unit (m, km, cm, mm, μm, nm, pm, ft, in, mi).
Enter the characteristic linear dimension (\( L \)) with its respective unit (m, km, cm, mm, μm, ft, in, mi).
Convert both \( \lambda \) and \( L \) to meters.
Calculate the Knudsen number using \( \text{Kn} = \frac{\lambda}{L} \).
Display the result, using scientific notation for values less than 0.001, otherwise with 4 decimal places.

3. Importance of Knudsen Number Calculation

Calculating the Knudsen number is crucial for:

Flow Regime Classification: Determining whether a gas flow behaves as a continuum (\( \text{Kn} \ll 1 \)) or requires statistical mechanics (\( \text{Kn} \gg 1 \)), affecting modeling in microfluidics and aerospace.
Design Applications: Guiding the design of systems like vacuum pumps, microchannels, and high-altitude aircraft where rarefied gas effects are significant.
Research: Assisting in studies of gas dynamics in low-pressure environments, such as in space or nanotechnology.

4. Using the Calculator

Examples:

Example 1: Calculate the Knudsen number for a gas with a mean free path of 100 nm and a characteristic length of 1 μm (e.g., a microchannel):
- Enter \( \lambda = 100 \) nm.
- Convert to m: \( \lambda = 100 \times 10^{-9} = 10^{-7} \, \text{m} \)
- Enter \( L = 1 \) μm.
- Convert to m: \( L = 1 \times 10^{-6} = 10^{-6} \, \text{m} \)
- \( \text{Kn} = \frac{10^{-7}}{10^{-6}} = 0.1 \)
- Result: \( \text{Kn} = 0.1000 \)
Example 2: Calculate the Knudsen number for a gas in a vacuum chamber where the mean free path is 500 pm and the characteristic length is 10 μm:
- Enter \( \lambda = 500 \) pm.
- Convert to m: \( \lambda = 500 \times 10^{-12} = 5 \times 10^{-10} \, \text{m} \)
- Enter \( L = 10 \) μm.
- Convert to m: \( L = 10 \times 10^{-6} = 10^{-5} \, \text{m} \)
- \( \text{Kn} = \frac{5 \times 10^{-10}}{10^{-5}} = 5 \times 10^{-5} \)
- Result: \( \text{Kn} = 5.0000e-5 \)

5. Frequently Asked Questions (FAQ)

Q: What does the Knudsen number tell us?
A: The Knudsen number indicates the flow regime of a gas. If \( \text{Kn} \ll 1 \), the flow is continuum; if \( \text{Kn} \gg 1 \), statistical mechanics (free molecular flow) applies.

Q: What is the characteristic linear dimension (\( L \))?
A: \( L \) is the minimum scale of length where significant differences in flow can be observed, such as the diameter of a pipe or the radius of a channel. Its definition may vary depending on the system geometry.

Q: Why must both inputs be positive?
A: Both the mean free path (\( \lambda \)) and characteristic length (\( L \)) are physical lengths and must be positive to have physical meaning.

Q: Why are smaller units like pm and nm useful for mean free path?
A: In rarefied gases or nanotechnology applications, the mean free path can be extremely small (on the order of nanometers or picometers), so these units allow for more precise input.