Ideal Gas Temperature Calculator

Pressure (\( P \)):

Volume (\( V \)):

Number of Moles (\( n \)):

1. What is the Ideal Gas Temperature Calculator?

Definition: This calculator computes the temperature (\( T \)) of an ideal gas based on its pressure (\( P \)), volume (\( V \)), and number of moles (\( n \)).

Purpose: It is used in physics and chemistry to determine the temperature of a gas under ideal conditions, aiding in thermodynamic analysis and gas law applications.

2. How Does the Calculator Work?

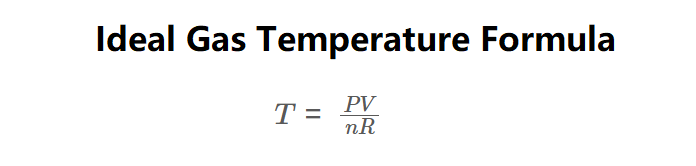
The calculator uses the ideal gas law rearranged for temperature:

\( T = \frac{PV}{nR} \)

Where:

\( T \): Temperature (K, °C, °F);
\( P \): Pressure (Pa, kPa, atm, bar);
\( V \): Volume (m³, L, cm³, ft³);
\( n \): Number of moles;
\( R \): Universal gas constant (\( 8.3145 \, \text{J·K}^{-1}\text{mol}^{-1} \)).

Steps:

Enter the pressure (\( P \)) with its unit.
Enter the volume (\( V \)) with its unit.
Enter the number of moles (\( n \)).
Convert pressure to Pa and volume to m³.
Calculate the temperature using \( T = \frac{PV}{nR} \).
Convert the result to the selected output unit.
Display the result, formatted in scientific notation if the absolute value is less than 0.001, otherwise with 4 decimal places.

3. Importance of Ideal Gas Temperature Calculation

Calculating the temperature of an ideal gas is crucial for:

Chemistry: Understanding gas behavior in reactions.
Physics: Studying thermodynamic processes.
Engineering: Designing systems involving gases, like engines or HVAC.

4. Using the Calculator

Example 1: Calculate the temperature with \( P = 1 \, \text{atm} \), \( V = 22.4 \, \text{L} \), \( n = 1 \, \text{mol} \):

Pressure: \( P = 1 \, \text{atm} \times 101325 = 101325 \, \text{Pa} \);
Volume: \( V = 22.4 \, \text{L} \times 0.001 = 0.0224 \, \text{m}^3 \);
Moles: \( n = 1 \, \text{mol} \);
Temperature: \( T = \frac{101325 \times 0.0224}{1 \times 8.3145} \approx 273.15 \, \text{K} \);
Result (in K): \( T = 273.1500 \, \text{K} \);
Result (in °C): \( T = 0.0000 \, \text{°C} \).

Example 2: Calculate the temperature with \( P = 1000 \, \text{Pa} \), \( V = 1 \, \text{m}^3 \), \( n = 0.1 \, \text{mol} \):

Pressure: \( P = 1000 \, \text{Pa} \);
Volume: \( V = 1 \, \text{m}^3 \);
Moles: \( n = 0.1 \, \text{mol} \);
Temperature: \( T = \frac{1000 \times 1}{0.1 \times 8.3145} \approx 1202.71 \, \text{K} \);
Result (in °F): \( T = (1202.71 - 273.15) \times 9/5 + 32 \approx 1701.08 \, \text{°F} \).

5. Frequently Asked Questions (FAQ)

Q: What is the ideal gas law?
A: The ideal gas law (\( PV = nRT \)) relates pressure, volume, number of moles, and temperature for an ideal gas, assuming no intermolecular forces.

Q: Why must temperature be positive?
A: The ideal gas law requires temperature in Kelvin, which must be positive (absolute zero is 0 K).

Q: Does this calculator apply to real gases?
A: This calculator uses the ideal gas law, which is an approximation. Real gases may deviate under high pressure or low temperature.