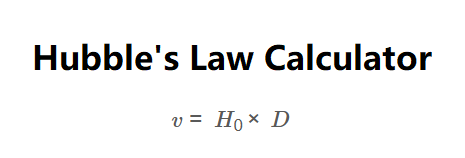1. What is Hubble's Law Calculator?
Definition: This calculator computes either the recessional speed (\( v \)) of a galaxy or its distance (\( D \)) from us, based on Hubble's Law, which describes the relationship between a galaxy's distance and its speed due to the expansion of the universe.
Purpose: It is used in cosmology to estimate distances to faraway galaxies or their recessional speeds, helping to understand the expansion rate of the universe.
2. How Does the Calculator Work?
The calculator uses the following formula:
Formula:
\[
v = H_0 \times D
\]
Where:
- \( v \): The speed of the galaxy (m/s, km/h, mph, km/s, mi/s, c)
- \( H_0 \): The Hubble constant (km/s/Mpc, km/s/kpc)
- \( D \): The distance to the galaxy (m, km, ft, mi, ly, au, pc, MLy, Mpc)
Unit Conversions:
- Hubble Constant (\( H_0 \)):
- 1 km/s/Mpc = 1 km/s/Mpc
- 1 km/s/kpc = 1000 km/s/Mpc (since 1 Mpc = 1000 kpc)
- Distance (\( D \)):
- 1 Mpc = 3.08568e22 meters
- 1 Mpc = 3.08568e19 km
- 1 Mpc = 1.01236e22 feet
- 1 Mpc = 1.91741e18 miles
- 1 Mpc = 3.262e6 light years
- 1 Mpc = 2.06265e11 AU
- 1 Mpc = 1e6 parsecs
- 1 Mpc = 3.262 MLy
- 1 Mpc = 1 Mpc
- Speed (\( v \)):
- 1 km/s = 1000 m/s
- 1 km/s = 3600 km/h
- 1 km/s ≈ 2236.936 mph (1 mph = 0.44704 m/s)
- 1 km/s = 1 km/s
- 1 km/s ≈ 0.621371 mi/s
- 1 km/s ≈ 3.33564e-6 c (c = 299792.458 km/s)
Steps:
- Select whether to calculate the speed (\( v \)) or the distance (\( D \)).
- Enter the Hubble constant (\( H_0 \)) with its unit (km/s/Mpc or km/s/kpc).
- Enter the known value (either \( D \) or \( v \)) with its unit.
- If calculating speed, select the desired result unit for \( v \).
- Convert all inputs to consistent units (km/s for speed, Mpc for distance, km/s/Mpc for \( H_0 \)).
- Calculate the unknown value using Hubble's Law.
- Convert the result to the selected unit if calculating speed.
- Display the result, using scientific notation for values less than 0.001, otherwise with 4 decimal places.
3. Importance of Hubble's Law Calculation
Calculating using Hubble's Law is crucial for:
- Measuring Cosmic Distances: It provides a method to estimate distances to galaxies based on their recessional speeds, which can be measured via redshift.
- Understanding Universe Expansion: The Hubble constant (\( H_0 \)) is a key parameter in cosmology, indicating the current rate of expansion of the universe.
- Cosmological Research: Helps in studying the large-scale structure of the universe and testing theories of cosmic evolution.
4. Using the Calculator
Examples:
- Example 1: Calculate the recessional speed of a galaxy at a distance of 1 Mpc, assuming a Hubble constant of 70 km/s/Mpc, with the result in km/s:
- Enter \( H_0 = 70 \) km/s/Mpc.
- Enter \( D = 1 \) Mpc.
- Select result unit as km/s.
- \( v = H_0 \times D = 70 \times 1 = 70 \) km/s
- Result: 70.0000 km/s
- Example 2: Calculate the same as above, but with the result in m/s:
- Enter \( H_0 = 70 \) km/s/Mpc.
- Enter \( D = 1 \) Mpc.
- Select result unit as m/s.
- \( v = 70 \) km/s
- Convert to m/s: \( 70 \times 1000 = 70000 \) m/s
- Result: 70000.0000 m/s
- Example 3: Calculate the distance to a galaxy with a recessional speed of 140 km/s, assuming a Hubble constant of 70 km/s/Mpc:
- Enter \( H_0 = 70 \) km/s/Mpc.
- Enter \( v = 140 \) km/s.
- \( D = \frac{v}{H_0} = \frac{140}{70} = 2 \) Mpc
- Result: 2.0000 Mpc
5. Frequently Asked Questions (FAQ)
Q: What is Hubble's Law?
A: Hubble's Law states that the recessional speed of a galaxy is proportional to its distance from us, with the Hubble constant (\( H_0 \)) as the proportionality factor. It is a key piece of evidence for the expansion of the universe.
Q: Why does the Hubble constant vary in different units?
A: The Hubble constant is typically given in km/s/Mpc, but can be expressed in other units like km/s/kpc for smaller distance scales, requiring conversion for consistency in calculations.
Q: Why is scientific notation used for some results?
A: For results less than 0.001, scientific notation is used to make extremely small values more readable.
Hubble's Law Calculator© - All Rights Reserved 2025
 Home
Home
 Back
Back