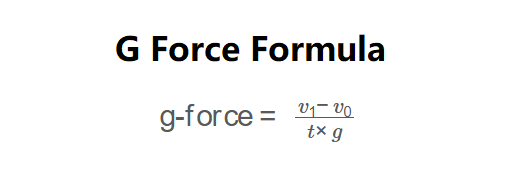G-Force Calculator

Initial Velocity (\( v_0 \)):

Final Velocity (\( v_1 \)):

Time (\( t \)):

1. What is the G-Force Calculator?

Definition: This calculator computes the g-force experienced during acceleration or deceleration, based on the initial velocity (\( v_0 \)), final velocity (\( v_1 \)), and time (\( t \)).

Purpose: It is used in physics, automotive safety, aerospace, and sports science to analyze the forces experienced during rapid changes in velocity.

2. How Does the Calculator Work?

The calculator uses the formula:

\( \text{g-force} = \frac{v_1 - v_0}{t \times g} \)

Where:

\( v_0 \): Initial velocity (m/s, km/h, ft/s, mph, kn, km/s);
\( v_1 \): Final velocity (m/s, km/h, ft/s, mph, kn, km/s);
\( t \): Time (sec, min, hrs, days, wks, mos, yrs);
\( g \): Acceleration due to gravity (\( 9.81 \, \text{m/s}^2 \));
\( \text{g-force} \): Dimensionless value representing acceleration in multiples of g.

Steps:

Enter the initial velocity (\( v_0 \)) with its unit.
Enter the final velocity (\( v_1 \)) with its unit.
Enter the time (\( t \)) with its unit.
Convert velocities to m/s and time to seconds.
Calculate the g-force using \( \text{g-force} = \frac{v_1 - v_0}{t \times g} \).
Display the result, formatted in scientific notation if the absolute value is less than 0.001, otherwise with 4 decimal places.

3. Importance of G-Force Calculation

Calculating g-force is crucial for:

Automotive Safety: Assessing crash forces for vehicle design.
Aerospace: Evaluating forces on pilots during takeoff or maneuvers.
Sports Science: Measuring forces experienced by athletes during high-speed activities.

4. Using the Calculator

Example 1: Calculate the g-force with \( v_0 = 60 \, \text{km/h} \), \( v_1 = 0 \, \text{km/h} \), \( t = 1 \, \text{sec} \):

Initial Velocity: \( v_0 = 60 \, \text{km/h} \times 0.277778 = 16.6667 \, \text{m/s} \);
Final Velocity: \( v_1 = 0 \, \text{km/h} = 0 \, \text{m/s} \);
Time: \( t = 1 \, \text{sec} \);
G-Force: \( \text{g-force} = \frac{0 - 16.6667}{1 \times 9.81} \approx -1.699 \);
Result: \( \text{g-force} = -1.6990 \).

Example 2: Calculate the g-force with \( v_0 = 0 \, \text{mph} \), \( v_1 = 100 \, \text{mph} \), \( t = 5 \, \text{min} \):

Initial Velocity: \( v_0 = 0 \, \text{mph} = 0 \, \text{m/s} \);
Final Velocity: \( v_1 = 100 \, \text{mph} \times 0.44704 = 44.704 \, \text{m/s} \);
Time: \( t = 5 \, \text{min} \times 60 = 300 \, \text{sec} \);
G-Force: \( \text{g-force} = \frac{44.704 - 0}{300 \times 9.81} \approx 0.0152 \);
Result: \( \text{g-force} = 0.0152 \).

5. Frequently Asked Questions (FAQ)

Q: What is g-force?
A: G-force is a measure of acceleration experienced by an object, expressed as a multiple of the acceleration due to gravity (\( g = 9.81 \, \text{m/s}^2 \)).

Q: Why can g-force be negative?
A: A negative g-force indicates deceleration (e.g., braking), where the final velocity is less than the initial velocity.

Q: Does this calculator account for air resistance?
A: No, this calculator assumes ideal conditions and does not account for external factors like air resistance.