Carnot Efficiency Calculator

1. What is the Carnot Efficiency Calculator?

Definition: This calculator computes the Carnot efficiency (\( \eta \)), which represents the maximum possible efficiency of a heat engine operating between two thermal reservoirs at temperatures \( T_h \) (hot) and \( T_c \) (cold), expressed in Kelvin.

Purpose: It is used in thermodynamics to determine the theoretical maximum efficiency of heat engines, such as those in power plants, refrigeration systems, and internal combustion engines.

2. How Does the Calculator Work?

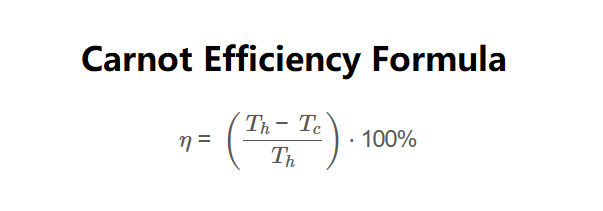
The calculator uses the Carnot efficiency formula:

Formula: \[ \eta = \left( \frac{T_h - T_c}{T_h} \right) \cdot 100\% \] where:

\( T_h \): Hot reservoir temperature (K, °C, °F)
\( T_c \): Cold reservoir temperature (K, °C, °F)
\( \eta \): Efficiency (%)

Unit Conversions:

Temperature (\( T_h \), \( T_c \)):
- Kelvin (K): No conversion needed
- Celsius (°C) to Kelvin: \( T_K = T_C + 273.15 \)
- Fahrenheit (°F) to Kelvin: \( T_K = (T_F - 32) \cdot \frac{5}{9} + 273.15 \)

Steps:

Enter the hot reservoir temperature (\( T_h \)) in K, °C, or °F (default is 500 K, step size 0.01).
Enter the cold reservoir temperature (\( T_c \)) in K, °C, or °F (default is 300 K, step size 0.01).
Convert both temperatures to Kelvin.
Calculate the efficiency as \( \eta = \left( \frac{T_h - T_c}{T_h} \right) \cdot 100\% \).
Display the result, using scientific notation if the absolute value is less than 0.001, otherwise rounded to 4 decimal places.

3. Importance of Carnot Efficiency Calculation

Calculating Carnot efficiency is crucial for:

Thermodynamic Limits: It provides the theoretical maximum efficiency for any heat engine, serving as a benchmark for real-world engines.
Engineering Design: It helps engineers optimize power plants, refrigeration systems, and other thermal machines by understanding efficiency limits.
Energy Research: It aids in developing more efficient energy conversion technologies, reducing energy waste.

4. Using the Calculator

Examples:

Example 1: Calculate the Carnot efficiency with a hot reservoir at 500 K and a cold reservoir at 300 K:
- Enter Hot Reservoir Temperature = 500 K.
- Enter Cold Reservoir Temperature = 300 K.
- Efficiency: \( \eta = \left( \frac{500 - 300}{500} \right) \cdot 100\% = 40\% \).
- Result: \( \eta = 40.0000\% \).
Example 2: Calculate the Carnot efficiency with a hot reservoir at 527 °C and a cold reservoir at 77 °F:
- Enter Hot Reservoir Temperature = 527 °C, convert to K: \( 527 + 273.15 = 800.15 \, \text{K} \).
- Enter Cold Reservoir Temperature = 77 °F, convert to K: \( (77 - 32) \cdot \frac{5}{9} + 273.15 = 298.15 \, \text{K} \).
- Efficiency: \( \eta = \left( \frac{800.15 - 298.15}{800.15} \right) \cdot 100\% \approx 62.7425\% \).
- Result: \( \eta = 62.7425\% \).

5. Frequently Asked Questions (FAQ)

Q: What is Carnot efficiency?
A: Carnot efficiency is the maximum possible efficiency of a heat engine operating between two thermal reservoirs, determined by the temperature difference between the hot and cold reservoirs.

Q: Why must temperatures be in Kelvin?
A: The Carnot efficiency formula requires absolute temperatures (Kelvin) because it is based on thermodynamic principles where temperature ratios matter, and Kelvin ensures positive values with a true zero point.

Q: What are some real-world applications of Carnot efficiency?
A: Carnot efficiency is used to evaluate the performance of power plants, design efficient refrigeration systems, and guide research in renewable energy technologies like solar thermal systems.