Capacitor Size Calculator

1. What is Capacitor Size Calculator?

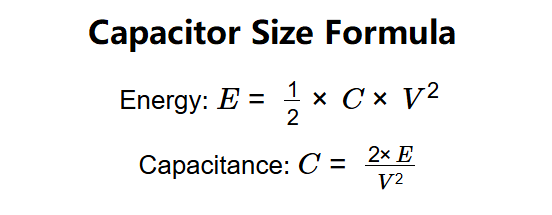
Definition: This calculator computes the required capacitance (\( C \)) for a capacitor to store a specific start-up energy (\( E \)) at a given voltage (\( V \)), often used in applications like electric motors.

Purpose: It is used in electronics to determine the appropriate capacitor size for energy storage, ensuring the capacitor can handle the required energy and voltage in circuits such as motor start-up circuits, power supplies, or defibrillators.

2. How Does the Calculator Work?

The calculator uses the energy storage formula for a capacitor:

Energy: \( E = \frac{1}{2} \times C \times V^2 \)
Capacitance: \( C = \frac{2 \times E}{V^2} \)

Where:

\( E \): Start-up energy (J)
\( V \): Voltage across the capacitor (V)
\( C \): Capacitance (F)

Steps:

Enter the start-up energy (\( E \)) and voltage (\( V \)) with their units.
Convert all inputs to base units (J, V).
Calculate the required capacitance using the formula.
Convert the result to the selected output unit.
Display the result with 4 decimal places.

3. Importance of Capacitor Size Calculation

Calculating the required capacitance is crucial for:

Motor Applications: Ensuring the capacitor can provide sufficient energy for motor start-up without exceeding voltage ratings.
Energy Storage: Designing circuits where capacitors store energy for applications like power backup or pulse circuits.
Safety: Selecting a capacitor with the appropriate capacitance and voltage rating to prevent overvoltage or failure.

4. Using the Calculator

Example 1: Calculate the required capacitance for a motor with a start-up energy of 0.06 J and a voltage of 20 V:

Start-up Energy (\( E \)): 0.06 J
Voltage (\( V \)): 20 V
Capacitance (\( C \)): \( \frac{2 \times 0.06}{20^2} = \frac{0.12}{400} = 0.0003 \, \text{F} = 300 \, \mu\text{F} \)
Result: \( C = 0.0003 \, \text{F} \) (or 300.0000 µF)

Example 2: Calculate the required capacitance for a circuit with a start-up energy of 50 mJ and a voltage of 100 V:

Start-up Energy (\( E \)): 50 mJ = 0.05 J
Voltage (\( V \)): 100 V
Capacitance (\( C \)): \( \frac{2 \times 0.05}{100^2} = \frac{0.1}{10000} = 0.00001 \, \text{F} = 10 \, \mu\text{F} \)
Result: \( C = 0.00001 \, \text{F} \) (or 10.0000 µF)

5. Frequently Asked Questions (FAQ)

Q: Why is capacitance important for motor start-up?
A: Capacitors in motors provide the necessary energy to overcome initial inertia during start-up. The capacitance must be sized correctly to store enough energy at the operating voltage.

Q: How does the dielectric material affect capacitance?
A: The dielectric material between the capacitor plates increases the capacitance by allowing more charge to be stored for the same voltage, depending on the material’s permittivity.

Q: What happens if the voltage exceeds the capacitor’s rating?
A: Exceeding the voltage rating can cause the capacitor to fail, potentially leading to dielectric breakdown, overheating, or even explosion in extreme cases.