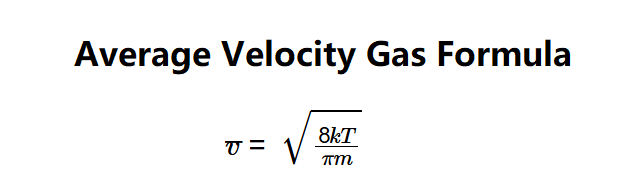Average Velocity of Gas Particles Calculator

Average Velocity of Gas Particles Formula

1. What is Average Velocity of Gas Particles Calculator?

Definition: This calculator computes the average velocity ( $\bar{v}$ ) of gas particles based on the Maxwell-Boltzmann distribution, which describes the speed distribution of particles in an ideal gas.

Purpose: It is used in physics and chemistry to understand the kinetic behavior of gas particles, which is essential for applications like gas dynamics, thermodynamics, and material science.

2. How Does the Calculator Work?

The calculator uses the Maxwell-Boltzmann formula for average velocity:

$\bar{v} = \sqrt{\frac{8 k T}{π m}}$

Where:

$m$ : Mass of a gas particle (kg);
$T$ : Temperature of the gas (K);
$k = 1.3806 \times 10^{- 23} J/K$ : Boltzmann constant;
$\bar{v}$ : Average velocity of the particles (m/s).

Steps:

Enter the temperature ( $T$ ) and its unit (K, °C, or °F).
Enter the mass of a gas particle ( $m$ ) and its unit (kg, g, or u).
Convert temperature to Kelvin and mass to kilograms.
Calculate the average velocity using the formula.
Convert the velocity to the selected output unit (m/s, km/h, or mph).
Display the result, formatted in scientific notation if the absolute value is less than 0.001, otherwise with 4 decimal places.

3. Importance of Average Velocity Calculation

Calculating the average velocity of gas particles is crucial for:

Gas Dynamics: Understanding the motion of gas particles, which affects diffusion, viscosity, and thermal conductivity.
Thermodynamics: Relating the kinetic energy of gas particles to temperature and pressure in the ideal gas law.
Chemical Reactions: Estimating collision rates between gas molecules, which influence reaction rates.

4. Using the Calculator

Example 1 (Nitrogen Gas at Room Temperature): Calculate the average velocity of nitrogen molecules:

Temperature: $T = 300 K$ ;
Mass of N₂ Molecule: $m = 28 u = 28 \times 1.66054 \times 10^{- 27} = 4.649512 \times 10^{- 26} kg$ ;
Average Velocity: $\bar{v} = \sqrt{\frac{8 \times (1.3806 \times 10^{- 23}) \times 300}{π \times (4.649512 \times 10^{- 26})}} \approx \sqrt{\frac{3.31344 \times 10^{- 20}}{1.460157 \times 10^{- 25}}} \approx \sqrt{226.929} \approx 476.585 m/s$ ;
Result: $\bar{v} = 476.5850 m/s$ .

Example 2 (Different Units): Calculate the average velocity with different units:

Temperature: $T = 77 °F$ ;
Mass of Gas Particle: $m = 4 \times 10^{- 26} kg$ ;
Convert temperature: $T = (77 - 32) \times 5 / 9 + 273.15 = 298.15 K$ ;
Average Velocity: $\bar{v} = \sqrt{\frac{8 \times (1.3806 \times 10^{- 23}) \times 298.15}{π \times (4 \times 10^{- 26})}} \approx \sqrt{\frac{3.292 \times 10^{- 20}}{1.256637 \times 10^{- 25}}} \approx \sqrt{261.936} \approx 511.794 m/s$ ;
Result in km/h: $\bar{v} = 511.794 \times 3.6 = 1842.4584 km/h$ .

5. Frequently Asked Questions (FAQ)

Q: What does the average velocity of gas particles represent?
A: The average velocity ( $\bar{v}$ ) represents the mean speed of gas particles in an ideal gas, derived from the Maxwell-Boltzmann distribution, reflecting their kinetic behavior at a given temperature.

Q: Why is the Boltzmann constant used?
A: The Boltzmann constant ( $k$ ) relates the temperature of the gas to the average kinetic energy of the particles, a fundamental constant in statistical mechanics.

Q: How does temperature affect the average velocity?
A: The average velocity is proportional to the square root of the temperature; as temperature increases, the particles move faster on average due to increased kinetic energy.