Absolute Value Calculator

1. What is the Absolute Value Calculator?

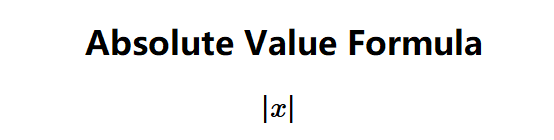
Definition: This calculator computes the absolute value of a number, which is the non-negative value of the number regardless of its sign. It represents the distance of the number from zero on a number line.

Purpose: It helps students, educators, and professionals quickly determine the absolute value of any number, useful in mathematics, physics, statistics, and more.

2. How Does the Calculator Work?

The calculator uses the following equation:

\[ |x| \]

Where:

\( x \): The input number;
\( |x| \): The absolute value, which is \( x \) if \( x \geq 0 \), or \( -x \) if \( x < 0 \).

Steps:

Enter the number (\( x \)).
Calculate the absolute value using the formula \( |x| \).
Display the result, formatted in scientific notation if the absolute value is less than 0.001, otherwise with 4 decimal places.

3. Importance of Absolute Value Calculation

Calculating the absolute value is crucial for:

Mathematics: Simplifies solving equations and inequalities involving absolute values.
Statistics: Used in measures like median absolute deviation to analyze data spread.
Physics: Represents magnitudes such as distance or speed, which are always non-negative.
Everyday Use: Useful in real-life scenarios like calculating differences without considering direction (e.g., temperature changes).

4. Using the Calculator

Example 1 (Positive Number): Calculate the absolute value of a positive number:

Input Number: \( x = 42 \);
Absolute Value: \( |42| = 42 \);
Result: \( |42| = 42.0000 \).

Example 2 (Negative Number): Calculate the absolute value of a negative number:

Input Number: \( x = -17.5 \);
Absolute Value: \( |-17.5| = 17.5 \);
Result: \( |x| = 17.5000 \).

5. Frequently Asked Questions (FAQ)

Q: What does the absolute value represent?
A: The absolute value of a number is its distance from zero on the number line, always a non-negative value. For example, \( |5| = 5 \) and \( |-5| = 5 \).

Q: Can the absolute value be negative?
A: No, the absolute value is always non-negative because it represents a distance, which cannot be negative.

Q: How is absolute value used in real life?
A: It’s used in scenarios like calculating the difference between two values (e.g., temperature changes, financial losses or gains) where the direction of change doesn’t matter, only the magnitude.