Cubic Yard from Square Feet Calculator

Area:

Depth / Height:

Price per Cubic Yard ($):

1. What is Cubic Yard from Square Feet Calculator?

Definition: This calculator computes the volume in cubic yards based on a given area (in square feet or other units) and depth, commonly used for estimating material needs in construction or landscaping projects. It also calculates the total cost based on the price per cubic yard.

Purpose: It helps users determine the amount of material (e.g., concrete, soil, gravel) required for a project when the base area and depth are known, and provides cost estimates to aid in budgeting.

2. How Does the Calculator Work?

The calculator uses the following equations to compute the volume and cost:

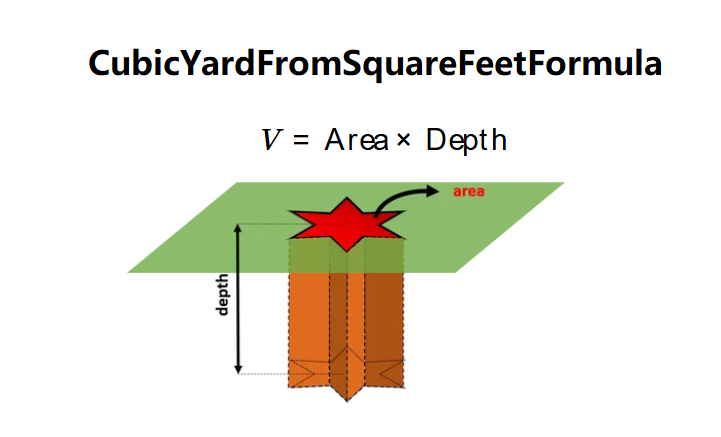
Volume: $ V = \text{Area} \times \text{Depth} $
Convert to Cubic Yards: $ V_{\text{yd}^3} = \frac{V_{\text{ft}^3}}{27} $
Cost: $ \text{Cost} = V_{\text{yd}^3} \times \text{Price per yd}^3 $

Where:

$ V $: Volume;
$ \text{Area} $: Base area of the surface (e.g., in square feet);
$ \text{Depth} $: Thickness or height of the material layer (e.g., in feet);
$ \text{Price per yd}^3 $: Cost of material per cubic yard.

Steps:

Enter the area of the surface and its unit (square feet, square inches, square yards, square meters, square centimeters, or square millimeters).
Enter the depth of the material layer and its unit (feet, inches, yards, meters, centimeters, or millimeters).
Enter the price per cubic yard for cost estimation.
Convert the area to square feet and depth to feet.
Calculate the volume in cubic feet by multiplying the area by the depth.
Convert the volume to cubic yards ($ V_{\text{ft}^3} / 27 $) or other selected units.
Calculate the total cost by multiplying the volume in cubic yards by the price per cubic yard.
Display results, formatted in scientific notation if the absolute value is less than 0.001, otherwise with 4 decimal places.

3. Importance of Cubic Yard from Square Feet Calculation

Calculating cubic yards from square feet is crucial for:

Construction Projects: Estimating the volume of concrete needed for slabs or footings when the area and thickness are known.
Landscaping: Determining the amount of mulch, soil, or gravel needed to cover a specific area to a certain depth.
Cost Efficiency: Ensuring accurate material estimates to avoid over-purchasing or under-purchasing, thus optimizing project budgets.

4. Using the Calculator

Example 1 (Concrete Slab): Calculate the volume and cost for a concrete slab with the following inputs:

Area: 100 ft²;
Depth: 6 in;
Price per Cubic Yard: $100;
Convert depth: $ 6 \, \text{in} = 6 / 12 = 0.5 \, \text{ft} $;
Volume in cubic feet: $ V = 100 \times 0.5 = 50 \, \text{ft}^3 $;
Volume in cubic yards: $ V = 50 / 27 \approx 1.8519 \, \text{yd}^3 $;
Cost: $ 1.8519 \times 100 \approx 185.19 \, \$ $;
Result: $ V = 1.8519 \, \text{yd}^3 $, Cost = $185.19.

Example 2 (Garden Mulch): Calculate the volume and cost for mulch in a garden bed:

Area: 50 m²;
Depth: 10 cm;
Price per Cubic Yard: $30;
Convert area: $ 50 \, \text{m}^2 \times 10.7639 = 538.195 \, \text{ft}^2 $;
Convert depth: $ 10 \, \text{cm} \div 30.48 \approx 0.3281 \, \text{ft} $;
Volume in cubic feet: $ V = 538.195 \times 0.3281 \approx 176.58 \, \text{ft}^3 $;
Volume in cubic yards: $ V = 176.58 / 27 \approx 6.5400 \, \text{yd}^3 $;
Cost: $ 6.5400 \times 30 \approx 196.20 \, \$ $;
Result: $ V = 6.5400 \, \text{yd}^3 $, Cost = $196.20.

5. Frequently Asked Questions (FAQ)

Q: What is a cubic yard?
A: A cubic yard is a unit of volume equal to the volume of a cube with sides of 1 yard (3 feet), or 27 cubic feet.

Q: How do I convert square feet and depth to cubic yards?
A: Multiply the area in square feet by the depth in feet to get the volume in cubic feet, then divide by 27 to convert to cubic yards.

Q: Why is this calculator useful for landscaping?
A: It simplifies calculating the volume of materials like mulch or soil needed to cover a known area to a specific depth, helping with accurate purchasing and cost estimation.