Potting Soil Calculator

Container Shape:

Length:

Width:

Depth:

1. What is the Potting Soil Calculator?

Definition: This calculator estimates the volume of potting soil needed to fill containers of different shapes (rectangular, round, or flower pot), based on the container's dimensions.

Purpose: It helps gardeners and landscapers determine the exact amount of potting soil required, ensuring proper planting conditions and efficient use of resources.

2. How Does the Calculator Work?

The calculator uses the following formulas based on the container shape:

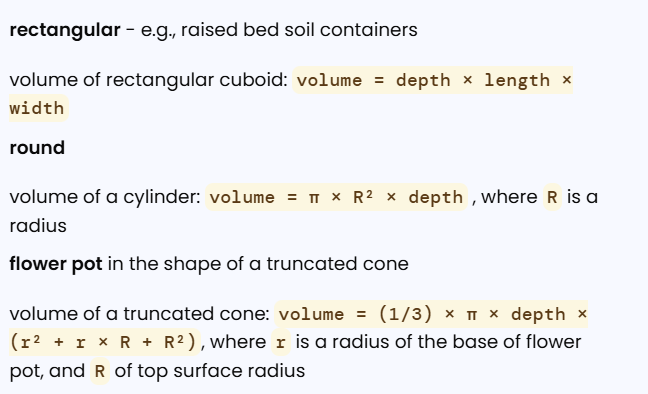
Rectangular (Raised Bed): \( \text{Volume} = \text{depth} \times \text{length} \times \text{width} \)
Round (Cylinder): \( \text{Volume} = \pi \times R^2 \times \text{depth} \), where \( R \) is the radius
Flower Pot (Truncated Cone): \( \text{Volume} = \frac{1}{3} \times \pi \times \text{depth} \times (r^2 + r \times R + R^2) \), where \( r \) is the base radius and \( R \) is the top radius

Steps:

Select the shape of your container (rectangular, round, or flower pot).
Enter the dimensions of the container and select their units (mm, cm, m, in, ft, yd).
Enter the depth of the container and its unit (mm, cm, m, in, ft, yd).
Convert each dimension to feet for calculation, then calculate the volume in cubic feet.
Convert the volume to the selected unit (ft³, yd³, m³, L).
Display the result as a numeric value, with the unit shown in the dropdown.

3. Importance of Potting Soil Calculation

Calculating the correct amount of potting soil is crucial for:

Plant Health: Ensures containers have enough soil for root growth and nutrient availability [Web ID: 0].
Cost Efficiency: Avoids over- or under-purchasing soil, saving money and preventing waste.
Planning: Helps plan for multiple containers or large gardening projects.

4. Using the Calculator

Example 1 (Rectangular Container, Mixed Units, Output in ft³): Calculate the potting soil needed for a rectangular raised bed:

Shape: Rectangular;
Length: 4 ft;
Width: 2 ft;
Depth: 6 in, convert to ft: \( 6 \div 12 = 0.5 \, \text{ft} \);
Volume: \( 4 \times 2 \times 0.5 = 4 \, \text{ft}^3 \);
Result: Potting Soil Needed = 4.0000 (unit: ft³).

Example 2 (Round Container, Metric Units, Output in L): Calculate the potting soil needed for a round container:

Shape: Round;
Radius: 30 cm, convert to ft: \( 30 \div 30.48 = 0.9843 \, \text{ft} \);
Depth: 40 cm, convert to ft: \( 40 \div 30.48 = 1.3123 \, \text{ft} \);
Volume: \( \pi \times 0.9843^2 \times 1.3123 \approx 3.1416 \times 0.969 \times 1.3123 \approx 3.9955 \, \text{ft}^3 \);
Convert to L: \( 3.9955 \times 28.3168 \approx 113.1596 \, \text{L} \);
Result: Potting Soil Needed = 113.1596 (unit: L).

Example 3 (Flower Pot, Mixed Units, Output in yd³): Calculate the potting soil needed for a flower pot (truncated cone):

Shape: Flower Pot;
Base Radius (r): 6 in, convert to ft: \( 6 \div 12 = 0.5 \, \text{ft} \);
Top Radius (R): 8 in, convert to ft: \( 8 \div 12 = 0.6667 \, \text{ft} \);
Depth: 12 in, convert to ft: \( 12 \div 12 = 1 \, \text{ft} \);
Volume: \( \frac{1}{3} \times \pi \times 1 \times (0.5^2 + 0.5 \times 0.6667 + 0.6667^2) \approx \frac{1}{3} \times 3.1416 \times (0.25 + 0.33335 + 0.44448) \approx 1.0472 \times 1.02783 \approx 1.0763 \, \text{ft}^3 \);
Convert to yd³: \( 1.0763 \div 27 \approx 0.0399 \, \text{yd}^3 \);
Result: Potting Soil Needed = 0.0399 (unit: yd³).

5. Frequently Asked Questions (FAQ)

Q: What depth should I use for potting soil?
A: The depth depends on the plant type, but typically ranges from a few inches for small plants to a foot or more for larger plants or raised beds. Check plant-specific recommendations for best results [Web ID: 0].

Q: Can I use different units for each dimension?
A: Yes, the calculator allows independent unit selection for each dimension (mm, cm, m, in, ft, yd). All measurements are converted to feet for calculation to ensure consistency.

Q: How do I convert soil volume from cubic feet to liters?
A: Multiply the volume in cubic feet by 28.3168 to get liters. For example, 1 ft³ = \( 1 \times 28.3168 = 28.3168 \, \text{L} \).